Estimate a phi function, and output omega

estRitaProperties(
  phidat,
  maxT,
  bigT,
  dt = 1/365.25,
  min_dt = FALSE,
  formula,
  family,
  use_geese,
  return_all = FALSE,
  plot_phi = FALSE,
  ...
)

Arguments

phidat: Data frame used to estimate the phi function, needs to have columns ri (recency indicator), ui (duration), and id (if use_geese == TRUE)
maxT: Maximum time to estimate phi until
bigT: T for estimating MDRI (what defines recent infection)
dt: An integration step size. Should be no more than 1 day.
min_dt: Make the minimum time dt (necessary if doing a log transformation of ui)
formula: Formula for fitting the model to phidat. Formula argument must take in only ui as the newdata. For example, do not create a ui^2 variable. Use poly(ui, ...) function to fit polynomial terms.
family: Family argument for glm or gee
use_geese: Indicator to fit a gee model using geese(), or a glm model with glm()
return_all: Whether to return additional objects used in internal functions (will use more memory)
plot_phi: Whether or not to plot the estimated phi function
...: Additional arguments to glm or geese for fitting model

Value

List of results:

omega: Estimate of Omega
omega_var: Variance of estimate of Omega
bigTidx: Index of bigT based on ts_index
ts_index: Index mapping each time point to an integer
get.integral.est: Function to give an estimate of Omega T for some T as arg to the function

Examples

# Define phi function
phi.func <- getTestRecentFunc(window=200, shadow=191)

# Simulate external study data
study <- simExternal(1, phi.func)
colnames(study) <- c("id", "ui", "ri")

# Estimate omega based on 3rd degree polynomial
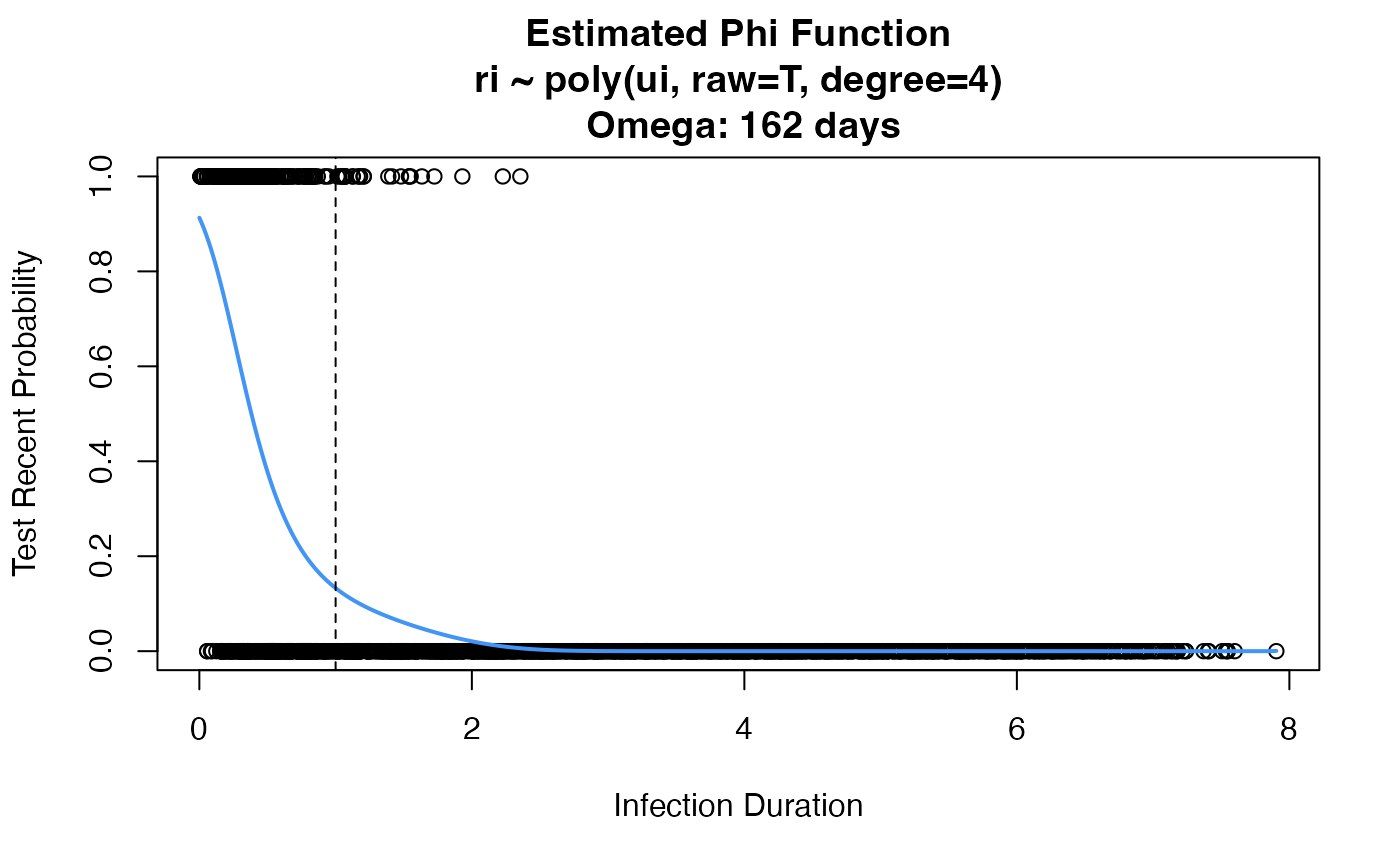
props <- estRitaProperties(
  phidat=study, maxT=5, bigT=1,
  formula="ri ~ poly(ui, raw=T, degree=4)", family=binomial(link="logit"),
  use_geese=TRUE, plot_phi=TRUE, return_all=TRUE)
#> Warning: glm.fit: fitted probabilities numerically 0 or 1 occurred


# Estimate omega based on 3rd degree polynomial, log function
# NOTE: If you do this, need to not have any ui = 0
study.log <- study
study.log[study.log$ui == 0, "ui"] <- 0.01
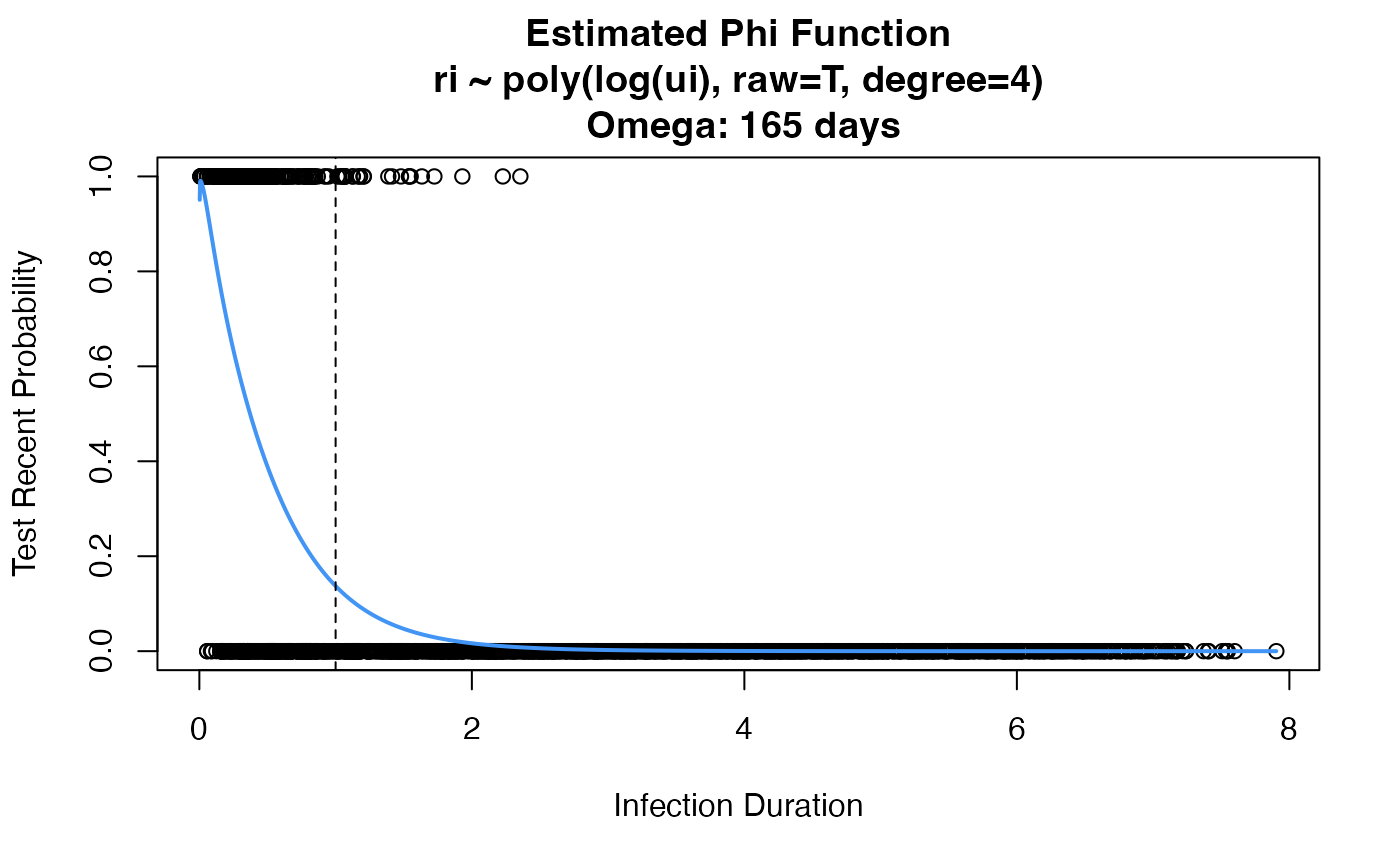
props <- estRitaProperties(
  phidat=study.log, maxT=10, bigT=1, min_dt=TRUE,
  formula="ri ~ poly(log(ui), raw=T, degree=4)", family=binomial(link="logit"),
  use_geese=TRUE, plot_phi=TRUE)


# Return additional function that can be used to
# quickly get other omega estimates
props <- estRitaProperties(
  phidat=study, maxT=5, bigT=1,
  formula="ri ~ poly(ui, raw=T, degree=3)", family=binomial(link="logit"),
  use_geese=TRUE, plot_phi=FALSE, return_all=TRUE)
#> Warning: glm.fit: fitted probabilities numerically 0 or 1 occurred

# What is \int_0^T \phi(t) dt for ts=T?
props$get.integral.est(ts=2.1)
#> $omega
#> [1] 0.5092978
#> 
#> $omega_var
#> [1] 0.0004007974
#> 
#> $idx
#> [1] 767
#>